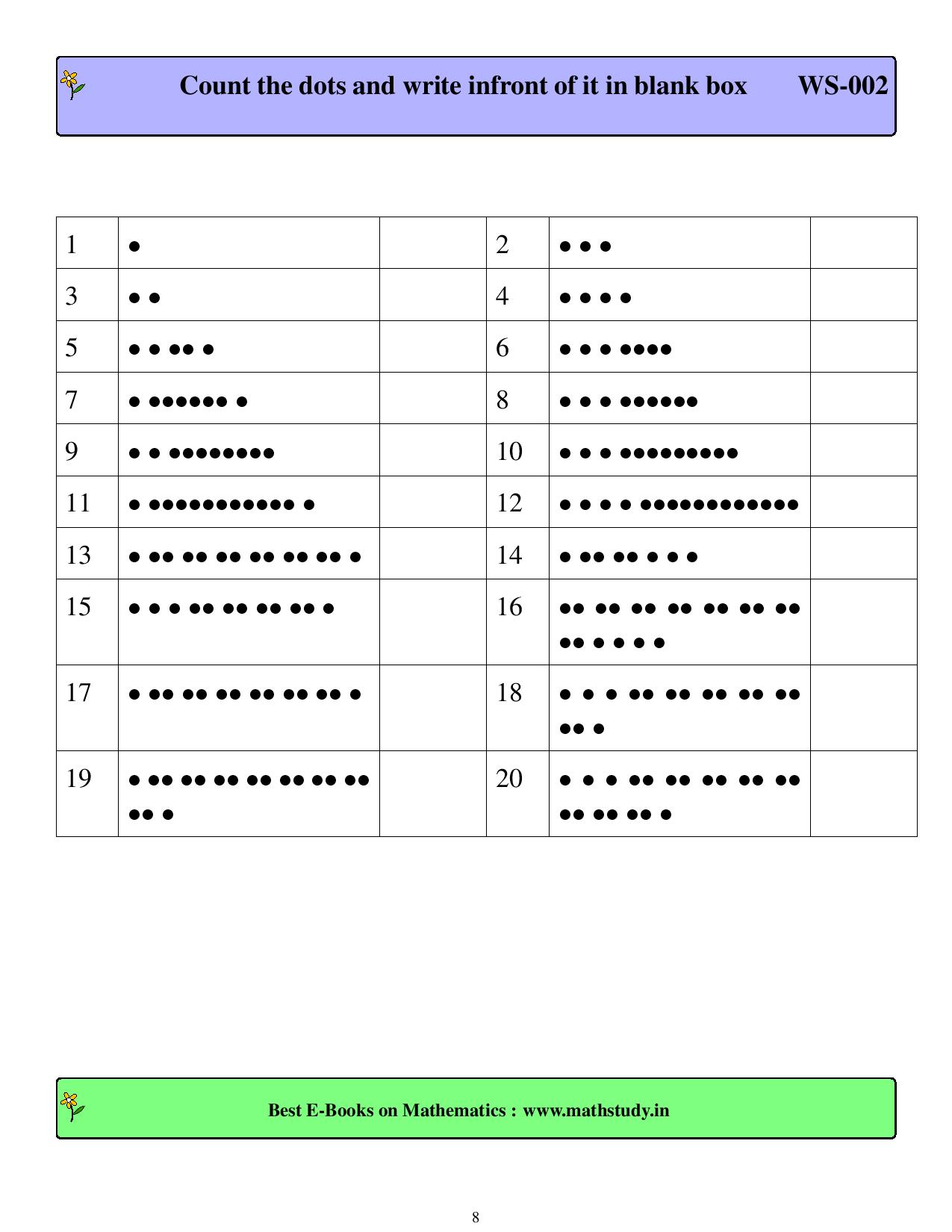
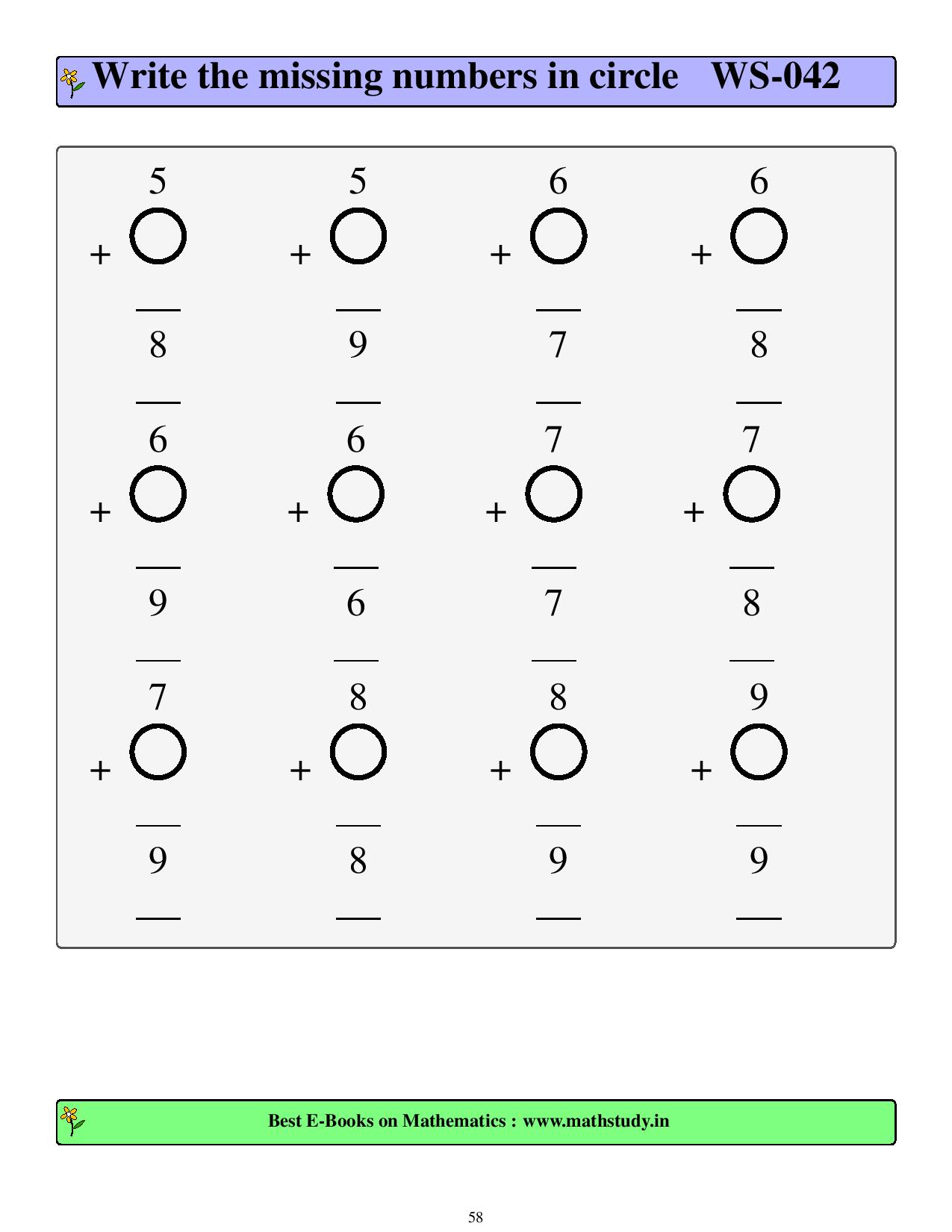
math workbook pdf grade 1 free download
math workbook pdf grade 1
math worksheet for class 1
worksheet class 1 maths
worksheet class 1 maths
If ${a_1},{a_2},{a_3}, \ldots ,{a_n}$ is an arithmetic progression with common difference
$d$, then evaluate the following Exemplarpression.
$\tan \left[ {{{\tan }^{ – 1}}\left( {\frac{d}{{1 + {a_1}{a_2}}}} \right) + {{\tan }^{ – 1}}\left( {\frac{d}{{1 + {a_2}{a_3}}}} \right) + {{\tan }^{ – 1}}\left( {\frac{d}{{1 + {a_3}{a_4}}}} \right)} \right.$ $\left. { + \ldots + {{\tan }^{ – 1}}\left( {\frac{d}{{1 + {a_{n – 1}}{a_n}}}} \right)} \right]$
We have, ${a_1} = a,{a_2} = a + d,{a_3} = a + 2d$and $d = {a_2} – {a_1} = {a_3} – {a_2} = {a_4} – {a_3} = \ldots = {a_n} – {a_{n – 1}}$