maths worksheet grade 1 chapter addition
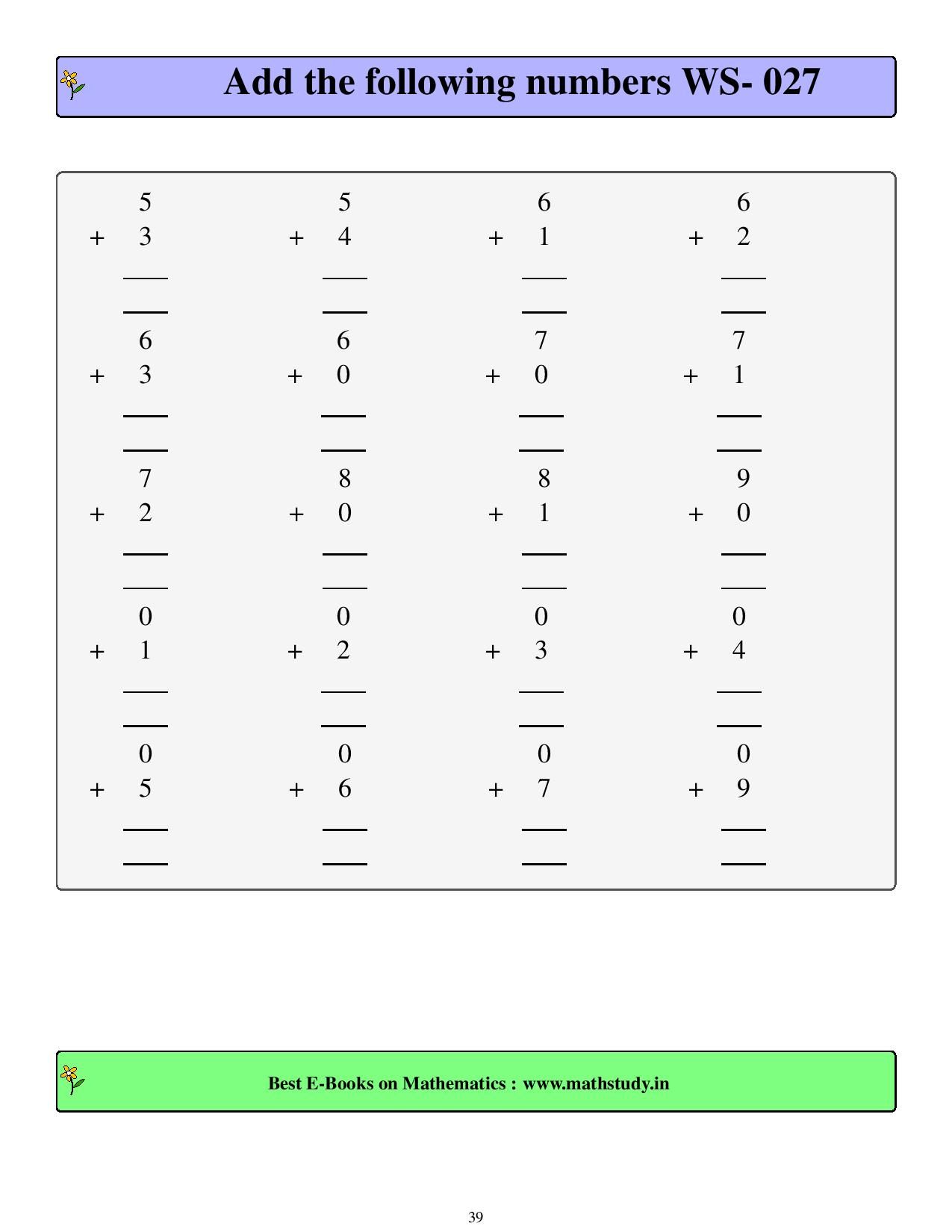
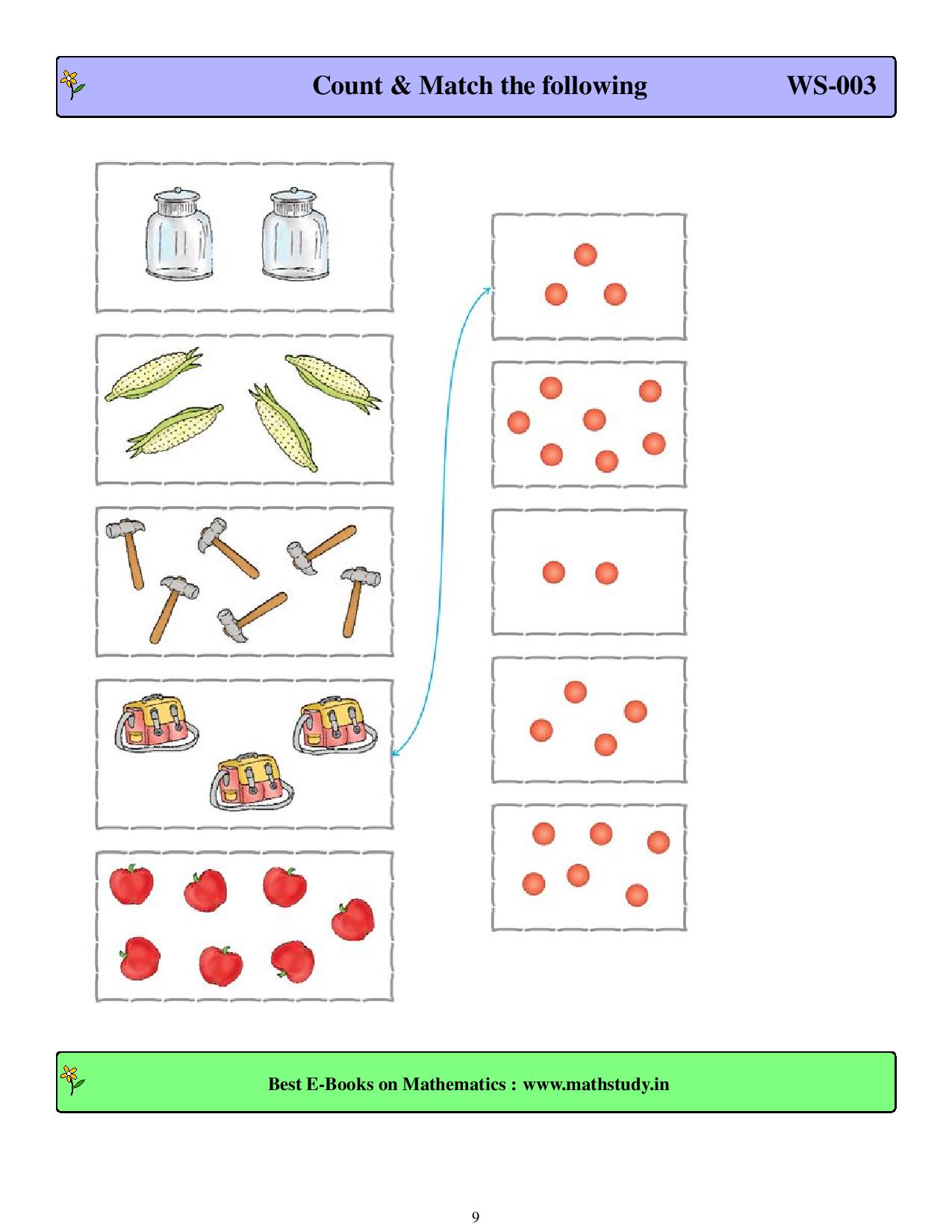
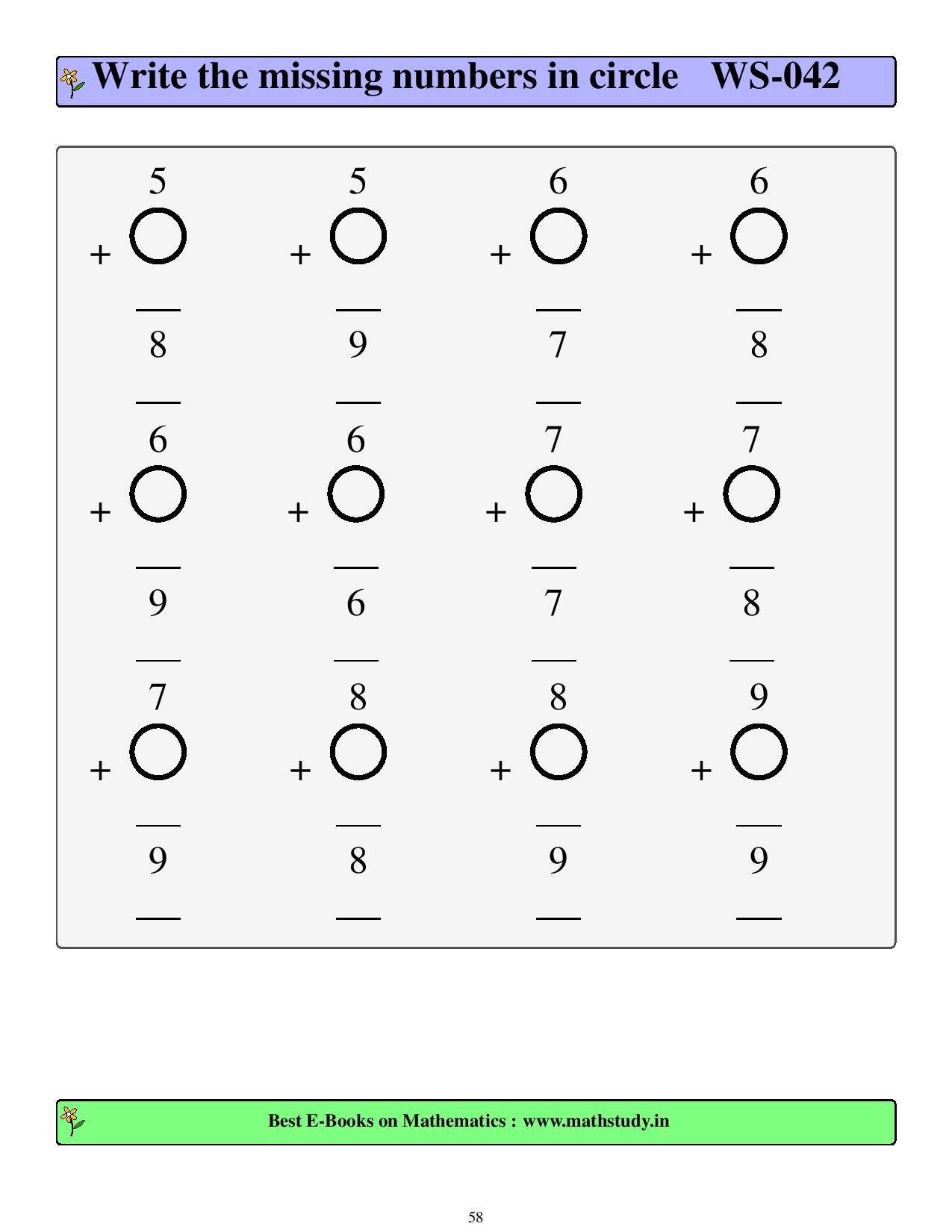
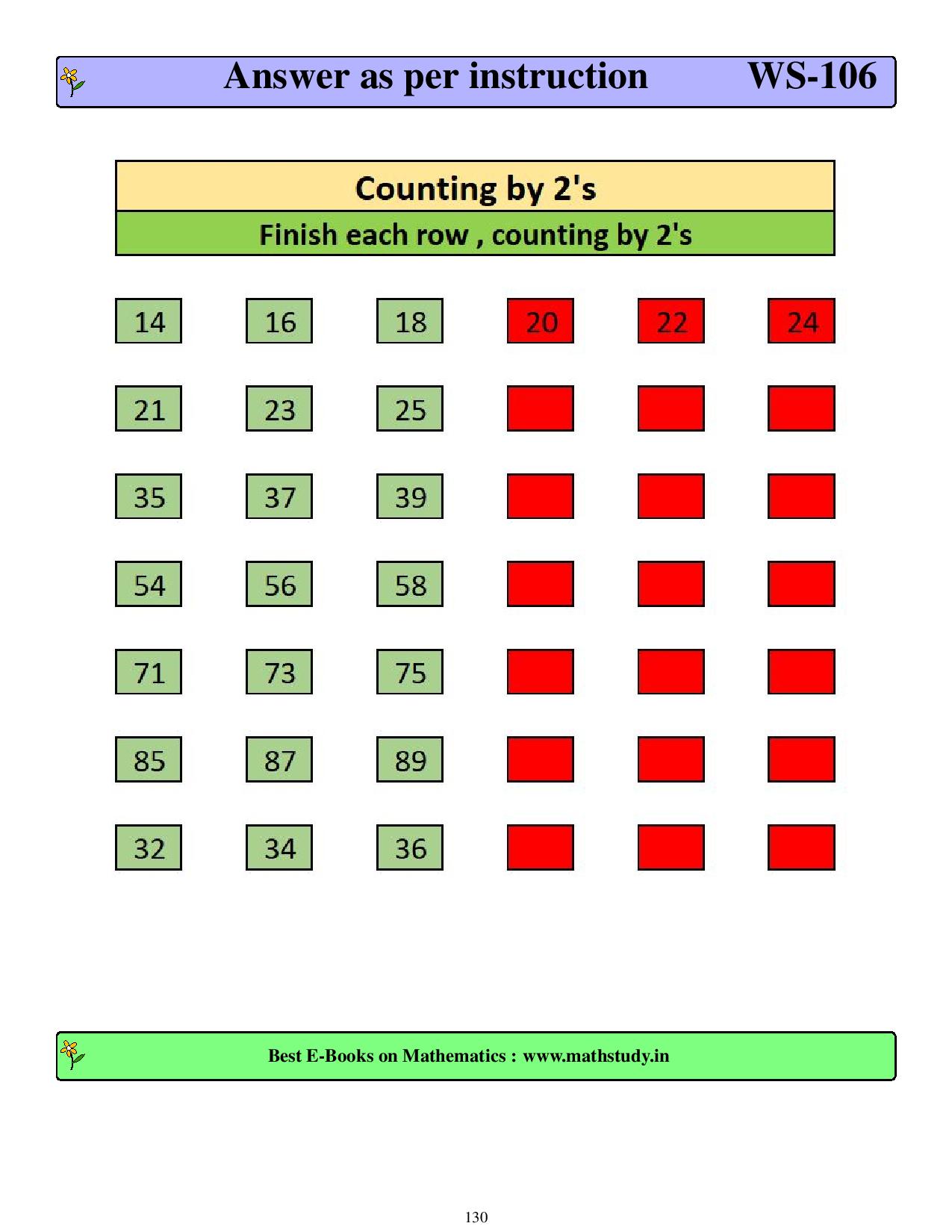
maths worksheet grade 1 chapter addition More than 150 worksheets. Worksheets on word problems which are fully solved HOTS(Higher Order Thinking Skills) questions are included to enhance the level of understanding. Help students to score in mathematics competition like International Mathematics Olympiad. Help student to develop analytical mind frame. Highly useful for those who want to learn programming language and conding. As per the international standard of curriculum and can be used by any student across the globe. Highly useful for parents,schools,teachers and coaching institutes.